Difference between revisions of "Modular model of the human CVS v.2"
Tagir Valeev (Talk | contribs) (DOI) |
|||
| (One intermediate revision by one user not shown) | |||
| Line 5: | Line 5: | ||
Complex modular model of the human CVS integrates three different models: | Complex modular model of the human CVS integrates three different models: | ||
| − | *[[Long-term model of the CVS/Renal system]] by Karaaslan et al<ref>Karaaslan F., Denizhan Y.,Kayserilioglu A., Gulcur H.O. Long-term mathematical model involving renal sympathetic nerve activity, arterial pressure, and sodium excretion // Ann. Biomed. Eng. 2005. Vol.33. P.1607 - 1630.</ref>. | + | *[[Long-term model of the CVS/Renal system]] by Karaaslan et al<ref>Karaaslan F., Denizhan Y.,Kayserilioglu A., Gulcur H.O. Long-term mathematical model involving renal sympathetic nerve activity, arterial pressure, and sodium excretion // Ann. Biomed. Eng. 2005. Vol.33. P.1607 - 1630. {{doi|10.1007/s10439-005-5976-4}}</ref>. |
*[[Short term model of the heart output regulation]] cerated by professor Soloyannikov<ref>Solodyannikov Y.V. The elements or mathematical modeling and hemodynamics system identification. Samara: The university of Samara, 1994.</ref>. | *[[Short term model of the heart output regulation]] cerated by professor Soloyannikov<ref>Solodyannikov Y.V. The elements or mathematical modeling and hemodynamics system identification. Samara: The university of Samara, 1994.</ref>. | ||
*[[1-dimensional arterial tree model]] | *[[1-dimensional arterial tree model]] | ||
| Line 13: | Line 13: | ||
Renal resistance to the arterial blood flow R<sub>r</sub> from the long-term CVS model was used for establishing of boundary conditions on the loose ends of renal arteries of the arterial tree model. | Renal resistance to the arterial blood flow R<sub>r</sub> from the long-term CVS model was used for establishing of boundary conditions on the loose ends of renal arteries of the arterial tree model. | ||
Renal afferent arterioles resistance Raa-ss was modeled as the value dependent from renal arteries resistance and passed to the long-term modules (after averaging to avoid systole-diastole oscillations) as well as renal blood flow Firb which was calculated as the outgoing blood flow from renal arteries. | Renal afferent arterioles resistance Raa-ss was modeled as the value dependent from renal arteries resistance and passed to the long-term modules (after averaging to avoid systole-diastole oscillations) as well as renal blood flow Firb which was calculated as the outgoing blood flow from renal arteries. | ||
| − | The created complex modular model comprises modules from three different models with different formalisms (ODE, PDE) and different time scales (seconds, minutes). The Model can be simulated using the [[agent-based approach]]. | + | The created complex modular model comprises modules from three different models with different formalisms (ODE, PDE) and different time scales (seconds, minutes). The Model can be simulated using the [[Agent based modeling|agent-based approach]]. |
==References== | ==References== | ||
Latest revision as of 11:40, 1 July 2013
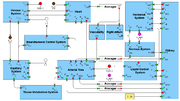
Complex modular model of the human CVS integrates three different models:
- Long-term model of the CVS/Renal system by Karaaslan et al[1].
- Short term model of the heart output regulation cerated by professor Soloyannikov[2].
- 1-dimensional arterial tree model
In the frames of the modular model, arterial tree is represented as a single module which input parameters are specified according to utilizing boundary conditions. Integration of the models was perfomed as follows: Arterial system module from the short-term CVS model was replaced by the arterial tree module. Blood flow from left ventricle was used to set boundary condition at the loose end of aorta,capillary resistance and venous pressure were used to establish filtration law at the loose ends of "last" vessels. Averaged parameters such as arterial total blood volume VA, outgoing blood flow from arteries QAC, arterial resistance RA were calculated in the arterial tree model and passed to the modules of the short-term CVS model. Renal resistance to the arterial blood flow Rr from the long-term CVS model was used for establishing of boundary conditions on the loose ends of renal arteries of the arterial tree model. Renal afferent arterioles resistance Raa-ss was modeled as the value dependent from renal arteries resistance and passed to the long-term modules (after averaging to avoid systole-diastole oscillations) as well as renal blood flow Firb which was calculated as the outgoing blood flow from renal arteries. The created complex modular model comprises modules from three different models with different formalisms (ODE, PDE) and different time scales (seconds, minutes). The Model can be simulated using the agent-based approach.
[edit] References
- ↑ Karaaslan F., Denizhan Y.,Kayserilioglu A., Gulcur H.O. Long-term mathematical model involving renal sympathetic nerve activity, arterial pressure, and sodium excretion // Ann. Biomed. Eng. 2005. Vol.33. P.1607 - 1630. doi:10.1007/s10439-005-5976-4
- ↑ Solodyannikov Y.V. The elements or mathematical modeling and hemodynamics system identification. Samara: The university of Samara, 1994.